Question

NO LINKS!! URGENT HELP PLEASE!!!

Find the area of ΔABC


5. c = 17, b = 13, a = 15


6. a = 23, b = 25, c = 14

Answer 1

5) 93.90 square units (2 d.p.)

6) 159.05 square units (2 d.p.)

Explanation:

To find the area of a triangle given its side lengths, use Heron's formula.

`\boxed{\begin{minipage}{8 cm}\underline{Heron's Formula}\\\\$A=√(s(s-a)(s-b)(s-c))$\\\\where:\\ \phantom{ww}$\bullet$ $A$ is the area of the triangle. \\ \phantom{ww}$\bullet$ $a, b$ and $c$ are the side lengths of the triangle. \\ \phantom{ww}$\bullet$ $s$ is half the perimeter.\\\end{minipage}}`

`\hrulefill`

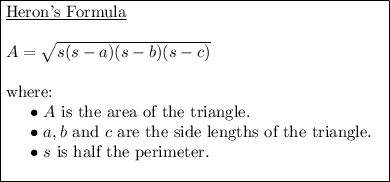
Question 5

Given side lengths of triangle ABC:

• a = 15
• b = 13
• c = 17

To find the half perimeter, s, half the sum of the 3 side lengths:

`\implies s=(a+b+c)/(2)=(15+13+17)/(2)=22.5`

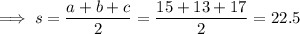
Substitute the values of a, b, c and s into Heron's formula and solve for area, A:

`\begin{aligned}A&=√(s(s-a)(s-b)(s-c))\\&=√(22.5(22.5-15)(22.5-13)(22.5-17))\\&=√(22.5(7.5)(9.5)(5.5))\\&=√(8817.1875)\\&=93.89988019...\\&=93.90\; \sf units^2\;(2\;d.p.)\end{aligned}`

Therefore, the area of triangle ABC is 93.90 square units, to two decimal places.

`\hrulefill`

Question 6

Given side lengths of triangle ABC:

• a = 23
• b = 25
• c = 14

To find the half perimeter, s, half the sum of the 3 side lengths:

`\implies s=(a+b+c)/(2)=(23+25+14)/(2)=31`

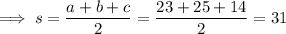
Substitute the values of a, b, c and s into Heron's formula and solve for area, A:

`\begin{aligned}A&=√(s(s-a)(s-b)(s-c))\\&=√(31(31-23)(31-25)(31-14))\\&=√(31(8)(6)(17))\\&=√(25296)\\&=159.0471628...\\&=159.05\; \sf units^2\;(2\;d.p.)\end{aligned}`

Therefore, the area of triangle ABC is 159.05 square units, to two decimal places.

Answer 2

5. 94 square units.

6. 159 square units.

Step-by-step explanation:

By using Heron's Formula:
`\bold{√(s(s-a)(s-b)(s-c))}`

5.

Using the values c = 17, b = 13, and a = 15,

Calculate the semi-perimeter of the triangle as:

s =
`(a+b+c)/(2)`

s =
`(15 + 13 + 17)/(2)=22.5`

Using Heron's formula, the area of the triangle is:

A =
`\bold{√(s(s-a)(s-b)(s-c))}`

A =
`√(22.5(22.5-15)(22.5-13)(22.5-17))=93.90` ≈94 approximately

Therefore, the area of triangle ABC is approximately 94 square units.

6.

Using the values, a = 23, b = 25, and c = 14,

we can calculate the semi-perimeter of the triangle as:

s =
`(a+b+c)/(2)`

s =
`( 23 + 25 + 14)/(2)`

s = 31

Using Heron's formula, the area of the triangle is:

A =
`\bold{√(s(s-a)(s-b)(s-c))}`

A =
`√(31(31-23)(31-25)(31-14))=159.05`≈159 approximately

Therefore, the area of triangle ABC is approximately 159 square units.