Question

Find the area of a horizontal cross section of a cylinder with a height of 34

centimeters and a circumference of about 131.88
centimeters. Use 3.14
for pie

Answer 1

• 1384.74 cm²

-------------------------

The circumference is given as 131.88 centimeters.

We know that the formula for the circumference is:

• C = 2πr, where r is the radius

So,

• 2πr = 131.88

Solve for radius:

• r = 131.88 / (2 × 3.14) = 21 cm

Use the area formula:

• A = πr²

Substitute 21 cm for r and calculate the area:

• A = 3.14 × 21² = 1384.74 cm²

Answer 2

1384.74 square centimeters

Explanation:

The area of a horizontal cross section of a cylinder is the same as the area of the circular base of the cylinder.

To find the area of the circular base of the cylinder, we first need to find the radius of the circle.

The formula for the circumference of a circle is C = 2πr, where r is the radius.

Given the circumference of the cylinder is 131.88 cm, and using 3.14 for π, we can use circumference formula to find the radius of the circular base:

`\begin{aligned}C &= 2\pi r\\\\\implies 131.88 &= 2 \cdot 3.14 \cdot r\\\\131.88 &= 6.28 r\\\\(131.88)/(6.28) &= (6.28 r)/(6.28)\\\\21&=r\\\\r&=21\; \sf cm\end{aligned}`

Substitute the found value of r into the formula for the area of a circle to find the area of a horizontal cross section of the cylinder:

`\begin{aligned}A &= \pi r^2\\\\A&=3.14 \cdot 21^2\\\\A&=3.14 \cdot 441\\\\A&=1384.74\;\sf cm^2\end{aligned}`

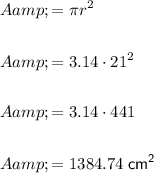
Therefore, the area of a horizontal cross section of the cylinder is approximately 1384.74 square centimeters.