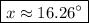Question

Need the answer to this using SOH-CAH-TOA

Answer 1

`x \approx 16.26\°`

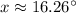
Explanation:

We can solve for angle x in this right triangle using the trigonometric ratio sine.

`\sin(\theta) = \frac{\text{opposite}}{\text{hypotenuse}}` ... This is the SOH part of SOH-CAH-TOA

↓ plugging in the given values

`\sin(x) = (7)/(25)`

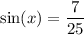
↓ taking the inverse sine of both sides

`\sin^(-1)(\sin(x)) = \sin^(-1)\left((7)/(25)\right)`

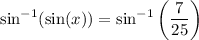
↓ canceling ...
`\sin^(-1)(\sin(\theta)) = \theta`

`x = \sin^(-1)\left((7)/(25)\right)`

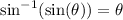
↓ plugging into a calculator

`\boxed{x \approx 16.26\°}`