Question

To approximate the length of a marsh , a surveyor walks x = 410 meters from point A to point B. Then , the surveyor turns and the length AC of the marsh. (Round your answer to one decimal place. ) 75 220 m

Answer 1

To find the length of AC, use the sine function with the given angle and the length of AB. Calculate sin(35) * 410 to find the approximate length of AC as 234.9 meters.

Step-by-step explanation:

To approximate the length of the marsh, the surveyor walks x = 410 meters from point A to point B. Then, the surveyor turns and measures the length AC of the marsh.

To find the length of AC, we need the length of AB and the angle BAC. Based on the information given, we can assume that AB is the baseline, and the angle BAC is 35 degrees.

Using trigonometry, we can use the sine function to find the length of AC. We have sin(35) = AC/AB. Substituting the values, we have sin(35) = AC/410. Solving for AC gives us AC = sin(35) * 410. Calculating this value gives us AC ≈ 234.9 meters (rounded to one decimal place).

Answer 2

Based on the calculations, an approximate length AC of the marsh is equal to 513.0 meters.

In Mathematics and Euclidean Geometry, the law of cosine can be represented by the following mathematical equation;

`C^2 = A^2 + B^2 - 2(A)(B)cos\theta`

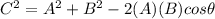
Where:

A, B, and C represent the side lengths of a triangle.

In order to determine an approximate length AC of the marsh, we would have to apply the law of cosine as follows:

`AC^2 = AB^2 + BC^2 - 2(AB)(BC)cos\theta\\\\AC^2 = 410^2 + 220^2 - 2(410)(220)cos(180 - 75)\\\\AC^2 = 168100 + 48400 - 2(410)(220)cos(105)\\\\AC^2 = 216500 - 180400(-0.25881904510252076234889883762405)\\\\AC^2 = 216500 +46690.955736494745527741350307378\\\\AC=√(263190.95573649474552774135030738)`

AC = 513.0214 ≈ 513.0 meters.

Complete Question:

To approximate the length of a marsh, a surveyor walks x = 410 meters from point A to point B, then turns 75 degrees and walks 220 meters to point C (see figure). Approximate the length AC of the marsh. (Round your answer to one decimal place. )