Answer:
d =
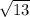
Explanation:
The formula for distance, d, between two points is
 , where (x1, y1) are one point and (x2, y2) are another point.
, where (x1, y1) are one point and (x2, y2) are another point.
We can allow (-6, 5) to be our (x1, y1) point and (-3, 7) to be our (x2, y2) point and plug the points into the formula:
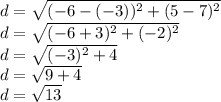
√13 is already in simplest radical form because there are no perfect squares which can be factored out from 13