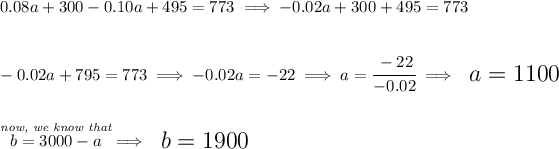Question

a person invested $6300 for 1 year part at 8% part at 10% and the remainder at 15%. The total annual income from these investments was $773. The amount of money invested at 15% was $300 more than the amounts invested at 8% and 10% combined. Find the amount invested at each rate.

Answer 1

a = amount invested at 8%

how much is 8% of "a"? (8/100) * "a", namely 0.08a.

b = amount invested at 10%

how much is 10% of "b"? (10/100) * "b", namely 0.10b.

c = amount invested at 15%

how much is 15% of "c"? (15/100) * "c", namely 0.15c.

we know the total amount invested is 6300, so whatever "a", "b" and "c" might be, we know that a + b + c = 6300.

we also know that the yielded amount in interest is 773, so if we simply add their interest, that'd be 0.08a + 0.10b + 0.15c = 773.

we also know that the combined amounts of "a + b" plus 300 bucks is "c", so really c = a + b + 300.

`c = a + b + 300 \\\\[-0.35em] ~\dotfill\\\\ a+b+c=6300\implies c=6300-a-b\implies \stackrel{\textit{substituting from the equation above}}{a+b+300=6300-a-b} \\\\\\ 2a+2b+300=6300\implies 2a+2b=6000\implies 2(a+b)=6000`

`a+b=\cfrac{6000}{2}\implies a+b=3000\implies \boxed{b=3000-a} \\\\[-0.35em] ~\dotfill\\\\ \stackrel{\textit{since we know that}}{c = a + b + 300}\implies \stackrel{\textit{substituting from above}}{c=a+(3000-a)+300}\implies {\Large \begin{array}{llll} c=3300 \end{array}} \\\\[-0.35em] ~\dotfill\\\\ \stackrel{ \textit{we also know that} }{0.08a+0.10b+0.15c=773}\implies \stackrel{\textit{now, substituting`

`0.08a+300-0.10a+495=773\implies -0.02a+300+495=773 \\\\\\ -0.02a+795=773\implies -0.02a=-22\implies a=\cfrac{-22}{-0.02}\implies {\Large \begin{array}{llll} a=1100 \end{array}} \\\\\\ \stackrel{\textit{now, we know that}}{b=3000-a}\implies {\Large \begin{array}{llll} b=1900 \end{array}}`

Answer 2

8%: $295

10% : $6005

15%: $595

Explanation:

1. The total invested amount was $6300
2. The total annual income from the investments was $773
3. The amount invested at 15% was $300 more than the amounts invested at 8% and 10% combined

Let's break this down:

Let's call the amounts invested at 8% and 10% combined = x
Then call the amount invested at 15% = x + $300
8% of x = 0.08x
10% of (6300 - x) = 0.1(6300 - x)
15% of (x + $300) = 0.15(x + $300)
0.08x + 0.1(6300 - x) + 0.15(x + $300) = $773
0.18x = $53
x = $53/0.18 = $295