Answer:
1.4 g Co(OH)₂
General Formulas and Concepts:
Math
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Chemistry
Unit 0
Atomic Structure
- Reading a Periodic Table
- Molarity = moles of solute / liters of solution
Aqueous Solutions
- States of Matter
- Prediction Reactions RxN
Stoichiometry
- Using Dimensional Analysis
- Analyzing Reactions RxN
Step-by-step explanation:
Step 1: Define
[RxN - Balanced] CoCl₂ + 2NaOH → 2NaCl + Co(OH)₂
[Given] 10.0 mL, 1.5 M CoCl₂
[Solve] grams Co(OH)₂
Step 2: Identify Conversions
[Base 10] 1000 mL = 1 L
[RxN] 1 mol CoCl₂ → 1 mol Co(OH)₂
[PT] Molar Mass of Co - 58.93 g/mol
[PT] Molar Mass of O - 16.00 g/mol
[PT] Molar Mass of H - 1.01 g/mol
Molar Mass of Co(OH)₂ - 58.93 + 2(16.00) + 2(1.01) = 92.95 g/mol
Step 3: Stoich
- [DA] Convert mL to L [Set up]:
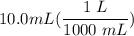
- [DA] Multiply/Divide [Cancel out units]:
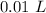
- [DA] Find moles of CoCl₂ [Molarity]:
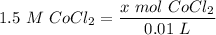
- [DA] Solve for x [Multiplication Property of Equality]:
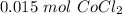
- [DA] Set up [Reaction Stoich]:
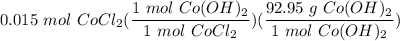
- [DA] Multiply/Divide [Cancel out units]:
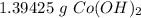
Step 4: Check
Follow sig fig rules and round. We are given 2 sig figs as our lowest.
1.39425 g Co(OH)₂ ≈ 1.4 g Co(OH)₂