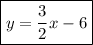Answer:
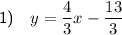
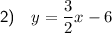
Explanation:
To find the equation of a line parallel to the line joining (1, 2) and (-2, -2) and passing through (4, 1), we first need to find the slope of the line joining (1, 2) and (-2, -2).
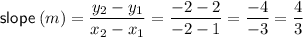
Parallel lines have the same slope, so the slope of the parallel line is m = 4/3.
Substitute the found slope and point (4, 1) into the point-slope formula:
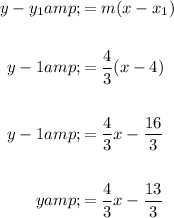
Therefore, the equation of the line parallel to the line joining (1, 2) and (-2, -2) and passing through (4, 1) is:
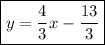

To find the equation of a line perpendicular to the line joining (2, -3) and (-1, -1) and passing through (2, -3), we first need to find the slope of the line joining (2, -3) and (-1, -1).
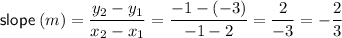
The slopes of perpendicular lines are negative reciprocals, so the slope of the parallel line is m = 3/2.
Substitute the found slope and point (2, -3) into the point-slope formula:
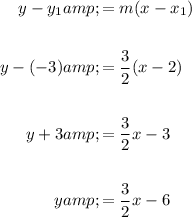
Therefore, the equation of the line perpendicular to the line joining (2, -3) and (-1, -1) and passing through (2, -3):