Question

Determine if the following are parallel perpendicular or neither

Answer 1

cheap answer, put them all in slope-intercept form, once we can see their slope, we know what's cooking.

same slope? likely parallel
different slope? likely neither

21)

we can rewrite them as

y = x + 6 and x +2 = y

slope in each is the same, 1, but the y-intercept differs, one is 6 the other 2, so they're parallel and a few units from each other.

22)

we can rewrite that as

`\cfrac{x-8}{2}=y\implies \cfrac{1}{2}x-4=y\hspace{7em}y=-2x+1`

so one has a slope of 1/2 and the other -2 hmmm, let's check for the negative reciprocal of 1/2

`\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{ \cfrac{1}{2}} ~\hfill \stackrel{reciprocal}{\cfrac{2}{1}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{2}{1} \implies -2}}`

ahaa!!, so one slope is the negative reciprocal of the other, they are perpendicular.

23)

`\cfrac{4x-9}{3}=y\implies \cfrac{4}{3}x-3=y\hspace{7em}\cfrac{3x-36}{4}=y\implies \cfrac{3}{4}x-9=y`

so one has a slope of 4/3 and the other of 3/4, neither.

24)

two horizontal lines, one above the other, parallel.

Answer 2

parallel, perpendicular, neither , parallel

Explanation:

• Parallel lines have equal slopes

• Product of slopes of perpendicular lines = - 1

the equation of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

21

y = x + 6 ← is in slope- intercept form , with slope m = 1

x - y = 2 ( subtract x from both sides )

- y = - x + 2 ( multiply through by - 1 )

y = x - 2 ← in slope- intercept form with slope = 1

Since the slopes are equal the lines are parallel

-----------------------------------------------------------------------------

22

x - 2y = 8 ( subtract x from both sides )

- 2y = - x + 8 ( divide through by - 2 )

y =
`(1)/(2)` x - 4 ← in slope- intercept form , with slope m =
`(1)/(2)`

y = - 2x + 1 ← in slope- intercept form, with slope m = - 2

then
`(1)/(2)` × - 2 = - 1

since the product is - 1 the lines are perpendicular

-----------------------------------------------------------------------------

23

4x + 3y = 9 ( subtract 4x from both sides )

3y = - 4x + 9 ( divide through by 3 )

y = -
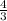`(4)/(3)` x + 3 ← in slope- intercept form , with slope = -
`(4)/(3)`

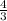
3x + 4y = 36 ( subtract 3x from both sides )

4y = - 3x + 36 ( divide through by 4 )

y = -
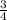`(3)/(4)` x + 9 ← in slope- intercept form, with slope m = -
`(3)/(4)`

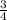
since slopes are neither equal nor product = - 1

The the lines are neither parallel nor perpendicular

-----------------------------------------------------------------------------

24

the equation of a horizontal line, parallel to the x- axis is

y = c ( c is the value of the y- coordinates the line passes through )

y = 5 and y = - 2 are both in this form , thus are parallel lines