Question

A ball of mass m = 0.275 kg swings in a vertical circular path tied to a string of L = 0.850 m long. (a) What are the forces acting on the ball at any point on the path? (b) Draw force diagrams for the ball when it is at the bottom of the circle and when it is at the top. (c) If its speed is 5.20 m/s at the top of the circle, what is the tension in the string there? (d) If the string breaks when its tension exceeds 22.5 N, what is the maximum speed the ball can have at the bottom before that happens?​

Answer 1

Given that a mass,
`\bold{m=0.275 \ kg}`, swings in a circular path due to an attached string with a length,
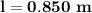`\bold{l=0.850 \ m}`. Refer to IMAGE #1 for a picture of the situation.

We are asked to answer the following...

(a) What forces always act on the mass throughout its swing?

(b) Draw force diagrams or free-body diagrams showing what forces are acting on the mass at the bottom of its swing vs the top.

(c) Given a velocity,
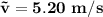`\bold{\vec v = 5.20 \ m/s}`, when the mass is at the top of its swing, find the tension in the string,
`\vec T`.

(d) Given the maximum tension,
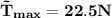`\bold{\vec T_(max)=22.5 N}`, what is the fastest the mass can travel at the bottom of its swing,
`\vec v_(max)`?

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

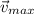For part (a):

To answer part a lets list of all forces that can act on an object and then narrow them down.

List of forces:

1. Weight or the force of gravity which is the mass of an object multiplied by the magnitude of the acceleration from gravity

(
`\vec w= m||\vec g|| \ where \ \vec g=-9.8 \ m/s^2`).

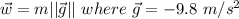2. Tension force which can be descried as a pulling force.

3. Normal force which is the force an object applies to prevent an object from traveling through its surface.

4. Friction force is a force that resists an objects motion.

5. Spring force is a force provided by a spring.

6. Air resistance force which is caused by air, can be interpreted as a friction force.

7. Electrical force is a force provided by charges.

8. Magnetic force which is a force provided by a magnetic field.

9. Buoyant force which is an upward force a fluid exherts on an object, can be interpreted as a normal force.

Right away we can eliminate 5,7,8, and 9. As we are not dealing with any springs, electricity, magnets, or fluids. We are also going to assuming there is no resistive forces acting on the ball so we can eliminate 4 and 6. The mass is not sitting on something so we can eliminate 3.

That leaves us with the gravitational force (weight) and the tension force. Which logically would make sense, gravity would act on the mass at all points in its path and the string will always provide a pulling force keeping the mass from unhooking and flying away in a straight line.

For part (b):

Refer to IMAGE #2

For part (c):

Refer to IMAGE #3

Add up forces acting in the centripetal axis. Note that the centripetal axis acts like the y axis and the tangential axis acts like the x axis.

`\vec v = 5.20 \ m/s`

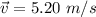
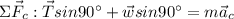`\Sigma \vec F_c: \vec Tsin90 \textdegree +\vec wsin90\textdegree=m\vec a_c` where
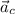`\vec a_c` is centripetal acceleration.
`\vec a _c = (\vec v^2)/(r)`

`\Longrightarrow \vec T(1) +\vec w(1)=m((\vec v^2)/(r) ) \Longrightarrow \vec T+\vec w=m((\vec v^2)/(r) ) \Longrightarrow \vec T=m((\vec v^2)/(r) ) -\vec w`

`\Longrightarrow \vec T=m((\vec v^2)/(r) ) -m ||\vec g|| \Longrightarrow \vec T=m(((\vec v^2)/(r) ) - ||\vec g||) \Longrightarrow \vec T=(0.275)((( (5.20)^2)/(0.850) ) - 9.8) \Longrightarrow \boxed{\boxed{\vec T = 6.05 N}} \therefore Sol.`

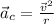
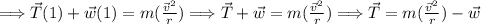
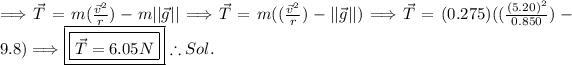
Thus, the tension in the string is found.

For part (d):

Refer to IMAGE #4

Add up forces acting in the centripetal axis. Note that the centripetal axis acts like the y axis and the tangential axis acts like the x axis.

`\vec T_(max)=22.5 \ N`

`\Sigma \vec F_c: \vec T_(max)sin90 \textdegree +\vec wsin90\textdegree=m\vec a_c`

`\Longrightarrow \vec T_(max)sin90 \textdegree +\vec wsin90\textdegree=m((v^2_(max))/(r) ) \Longrightarrow \vec T_(max)(1) +\vec w(1)=m((v^2_(max))/(r) )`

`\Longrightarrow \vec T_(max) +\vec m||\vec g||=m((v^2_(max))/(r) ) \Longrightarrow \vec v_(max)= \sqrt)/(m))`

`\Longrightarrow \vec v_(max)= \sqrt{(0.850)((22.5+(0.275)(9.8))/(0.275)}) \Longrightarrow \boxed{\boxed{\vec v_(max)=8.82 \ m/s} } \therefore Sol.`

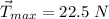
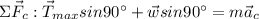
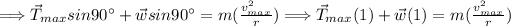
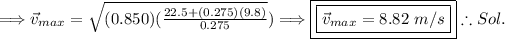
Thus, the max speed is found.