Question

I have three math questions

Decide whether the given ordered pair is a solution to the system of linear inequalities

1 - y > x - 6

y < x -1

(5,2)



2 - y (< with a line under it i dont know how to type it) 2x

y (> with a line under it) x

(-3, -6)



3 - (1 over 2)x +3y < 8

y (> with a line under it) 1

(0, (2 over 3) )

Answer 1

1. Not true, so (5,2) is not a solution

2. Not true, so (-3,-6) is not a solution.

3. Not true, so (0,
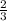`(2)/(3)`) is not a solution.

Explanation:

Substitute 5 for x and 2 for y

1 - y > x - 6

1 - 2 > 5 - 6

-1 > -1

This is not true. -1 is not greater than -1

y < x - 1

Substitute -3 for x and -6 for y

2 - y
`\leq` 2x

2 - (-6)
`\leq` 2(-3)

8
`\leq` -6

This is not true. 8 is not less than -6.

y
`\geq` x

Substitute 0 for x and
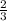
`(2)/(3)`

3 -
`(1)/(2)` x + 3y < 8

3 -
`(1)/(2)` (0) + 3(
`(2)/(3)`) < 8

3 - 0 +
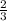`(6)/(3)` < 8

3 + 2 < 8

6 < 8

This is true. 6 is less than 8

y
`\geq` 1

`(2)/(3)`
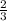
`\geq` 1

This is not true.
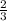`(2)/(3)` is not greater than or equal to one.

The ordered pair has to be a solution for both equations for the ordered pair to be a solution for the system.

Helping in the name of Jesus.

Answer 2

1. To check if (5,2) is a solution, we need to substitute x=5 and y=2 into both inequalities and check if both inequalities are true:

- y > x - 6 ==> 2 > 5 - 6 ==> 2 > -1 (true)
- y < x - 1 ==> 2 < 5 - 1 ==> 2 < 4 (true)

Both inequalities are true when x=5 and y=2, so (5,2) is a solution to the system of linear inequalities.

2. To check if (-3,-6) is a solution, we need to substitute x=-3 and y=-6 into both inequalities and check if both inequalities are true:

- y ≤ 2x ==> -6 ≤ 2(-3) ==> -6 ≤ -6 (true)
- y ≥ x ==> -6 ≥ -3 (false)

The second inequality is not true when x=-3 and y=-6, so (-3,-6) is not a solution to the system of linear inequalities.

3. To check if (0, 2/3) is a solution, we need to substitute x=0 and y=2/3 into both inequalities and check if both inequalities are true:

- (1/2)x + 3y < 8 ==> (1/2)(0) + 3(2/3) < 8 ==> 2 < 8 (true)
- y > 1 ==> 2/3 > 1 (false)

The second inequality is not true when x=0 and y=2/3, so (0, 2/3) is not a solution to the system of linear inequalities.