Question

A storage tank has the shape of an inverted circular cone with height 12 m and base radius of 4 m. It is filled with water to a height of 10 m. Find the work required to empty the tank by pumping all of the water to the top of the tank. (The density of water is 1000 kg/m3. Assume g

Answer 1

Work required to empty the tank by pumping all of the water to the top of the tank = 1674700 Kgm/s^2

Step-by-step explanation:

Volume of Circular cone = V = (1/3)πr2h

where r is the radius in meters

and h is the height in meters

Substituting the given values in above equation, we get -

V =
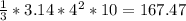`(1)/(3) * 3.14 * 4^2 * 10 = 167.47` cubic meters.

The force required will be equal to the mass of water in the cone

`= 167.47 * 1000`

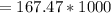
= 167470 Kg

Weight = Mass * g

= 167470 * 10

= 1674700 Kgm/s^2