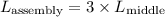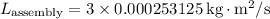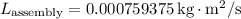(a) The rotational inertia of the assembly is
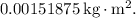
(b) The magnitude of the angular momentum of the middle particle is
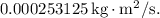
(c) The magnitude of the angular momentum of the assembly is
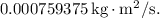
(a) The rotational inertia of the assembly:
The formula for the rotational inertia of point masses rotating about an axis is
 is the mass of each point particle and
is the mass of each point particle and
 is its distance from the axis of rotation.
is its distance from the axis of rotation.
Given that the mass of each particle is
 and the length of each rod is
and the length of each rod is
 , the distance of each particle from the axis of rotation is
, the distance of each particle from the axis of rotation is
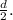
The rotational inertia for each particle about point O is

Since there are three particles, the total rotational inertia of the assembly is
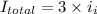
Plug in the values to find
 :
:
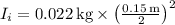
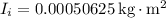
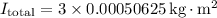

(b) The magnitude of the angular momentum of the middle particle:
The formula for angular momentum is
 , where I is the rotational inertia and
, where I is the rotational inertia and
 is the angular speed.
is the angular speed.
For the middle particle, the rotational inertia is \( I_i = m \cdot \left(\frac{d}{2}\right)^2 \) as calculated before.
Angular momentum of the middle particle
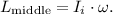
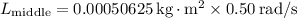
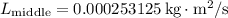
(c) The magnitude of the angular momentum of the assembly:
Since the three particles rotate together, the angular momentum of the assembly is the sum of the angular momenta of all three particles.