Question

Charlotte, a 45 kg deep diver, shoots a 2 kg spear with a speed of 15 m/s at a fish that darts away without getting hit. How fast does Charlotte move backwards when the spear is shot?

Answer 1

-0.67 m/s

Step-by-step explanation:

This problem can be solved using the principle of conservation of momentum.

The initial momentum of the system is 0 because both Charlotte and the spear are at rest.

Let’s denote Charlotte’s velocity after the spear is shot as v. The final momentum of the system is given by the sum of the momenta of Charlotte and the spear: (45 kg) * v + (2 kg) * (15 m/s).

By the principle of conservation of momentum, the initial and final momenta of the system must be equal. Therefore, we have:

(45 kg) * v + (2 kg) * (15 m/s) = 0

Solving for v, we find that:

v = -(2 kg * 15 m/s) / (45 kg)

v ≈ -0.67 m/s

So Charlotte moves backward with a velocity of approximately -0.67 m/s.

Answer 2

Approximately
`(-0.67)\; {\rm m\cdot s^(-1)}` assuming that Charlotte was initially not moving.

Step-by-step explanation:

When an object of mass
`m` travels at a velocity of
`v`, the momentum
`p` of that object would be
`p = m\, v`.

Assuming that Charlotte was initially not moving, the momentum of Charlotte and the spear would both be
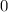`0` before launching the spear.

At a velocity of
`15\; {\rm m\cdot s^(-1)}`, the momentum of the
`2\; {\rm kg}` spear would be
`(2\; {\rm kg})\, (15\; {\rm m\cdot s^(-1)}) = 30\; {\rm kg \cdot m\cdot s^(-1)}` after the launch.

If the velocity of Charlotte after launching the spear is
`v\; {\rm m\cdot s^(-1)}`, the momentum of Charlotte would be
`(45)\, v\; {\rm kg\cdot m\cdot s^(-1)}`.

Momentum is supposed to be conserved immediately launching the spear. In other words, the sum of the momentum of Charlotte and the spear should be the same before and after before launching the spear:

• Total momentum before launching the spear:
  `0\; {\rm kg\cdot m\cdot s^(-1)}`.
• Total momentum after launching the spear:
  `(45\, v + 30)\; {\rm kg\cdot m\cdot s^(-1)}`.

By the conservation of momentum:

`45\, v + 30 = 0`.

`v \approx (-0.67)`.

In other words, the speed of Charlotte would be approximately
`(-0.67)\; {\rm m\cdot s^(-1)}` immediately after launching the spear.