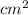Question

A rectangle has a length of x + 4 cm and a width of 2x − 7 cm.

(a) If the perimeter is 36cm, what is the value of x?
(b) What is the area of this rectangle

Answer 1

Answer: (a) x=7

(b) area of this rectangle = 77
`cm^(2)`

Explanation:

(a) the perimeter of a rectangle = 2 * [ l + b ]

36 = 2 * [ (x + 4) + (2x - 7) ]

36 / 2 = x + 4 + 2x - 7

18 = 3x -3

18 + 3 = 3x

21 = 3x

x = 7

(b) the area of a rectangle = l * b

l = x + 4 = 11cm

b = 2x -7 = 7cm

therefore, 11cm * 7cm = 77
`cm^(2)`

Answer 2

See below!

Explanation:

Given data:

Length = x + 4 cm

Width = 2x - 7 cm

Part a)

Perimeter:

• Sum of all sides of a shape is called perimeter.

Perimeter of rectangle = 2(length) + 2(width)

Put the given data.

36 = 2(x + 4) + 2(2x - 7)

• Distribute

36 = 2x + 8 + 4x - 14

• Combine like terms

36 = 2x + 4x + 8 - 14

36 = 6x - 6

• Add 6 to both sides

36 + 6 = 6x

42 = 6x

• Divide both sides by 6

42/6 = x

7 = x

OR

x = 7

Part b)

Area of rectangle = length × width

Area = (x + 4)(2x - 7)

Put x = 7

Area = (7 + 4)(2(7) - 7)

Area = 11(14 - 7)

Area = 11(7)

Area = 77 cm²

`\rule[225]{225}{2}`