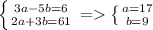Question

The area of a rectangle gets reduced by 9 square units if its length is reduced by 5 units and the breadth is increased by 3 units. If we increase the length by 3 units and breadth by 2 units, th

e area is increased by 67 square units. Find the length and breadth of the rectangle.

Answer 1

length = 17; breadth = 9

Explanation:

Let x = area of rectangle; a = length; b = breadth.

+) a × b = x (1)

+) (a - 5) × (b + 3) = x - 9 => x = ab + 3a - 5b - 15 + 9 (2)

+) (a + 3) × (b + 2) = x + 67 => x = ab + 2a + 3b + 6 - 67 (3)

Replace (1) into (2) & (3):

`\left \{ {{3a - 5b=6} \atop {2a+3b=61}} \right. = > \left \{ {{a=17} \atop {b=9}} \right.`