Question

A boat traveled 350 miles each way downstream and back. The trip downstream took 14 hours.

The trip back took 70 hours. What is the speed of the boat in still water? What is the speed of
the current?

Answer 1

The speed of the boat in still water is 15 mi/hr while the speed of the current is 10 mi/hr.

Explanation:

Given: d = 350 miles

`t_(ds)` = 14 hours

`t_(us)` = 70 hours

Asked: Speed of the boat
`V_b` in still water and speed of the current
`V_c`

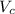
Solve:

Note that when the boat is going downstream, the current is helping the boat to speed up and when the boat is going upstream, the current is slowing it down.

Get the speed downstream

`V_(ds) = V_b + V_c`

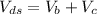
350mi/14hrs =
`V_b + V_c`

25 mi/hr = 
`V_b + V_c`

Transpose to get the value of Vb

25 mi/hr - Vc = Vb ---equation 1

Get the speed upstream

`V_(us) = V_b - V_c`

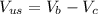
350mi/70hrs = Vb - Vc

5 mi/hr = Vb - Vc

Transpose to get the value of Vb

5 mi/hr + Vc = Vb ----equation 2

Equate equations 1 and 2

25 mi/hr - Vc = 5 mi/hr + Vc

Transpose

25 mi/hr - 5 mi/hr = Vc + Vc

20 mi/hr = 2 Vc

Divide both sides of the equation by 2

10 mi/hr = Vc

Vc = 10 mi/hr

From 25 mi/hr = 
`V_b + V_c` we then solve the speed of the boat

25 mi/hr = Vb + 10 mi/hr

25 mi/hr - 10 mi/hr = Vb

15 mi/hr = Vb

Vb = 15 mi/hr