Question

3. Soshi's rhombus has a base of 12 in. and a

height of 10 in. Jack's rhombus has base and
height measures that are double those of Soshis
rhombus. Compare the area of Jack's rhombus to
the area of Soshi's rhombus.

Answer 1

The area of Jack's rhombus is four times the area of Soshi's rhombus.

Explanation:

First, calculate the area of Soshi's rhombus. Use the formula for the area of a rhombus:

`A = bh=\\A=(12)(10)=\\A=120`

Now, we have the Soshi's rhombus is 120
`in^2`.

Jack's rhombus' base and height are double those of Soshi's; multiply each value by two.

`12*2=24\\\\10*2=20`

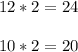
Now, substitute the values in the formula for the area of a rhombus:

`A = bh=\\A=(24)(20)=\\A=480`

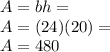
Notice that 480 is 4 times 120.

This is because area is a two-dimensional measure (measured in units squared, like
`in^2`) while length is a one-dimensional measure (measured in regular units, like cm and in), The one-dimensional measures of Jack's rhombus are double those of Soshi's, and to translate this to the two-dimensional measure of area, square 2 (2 squared is 2 times 2).

2 squared is 4, so:

the area of Jack's rhombus is four times that of Soshi's.