Question

Find the value of x in the triangle shown below.

X=
4.5
56°
4
4
X°

Answer 1

68.9 degrees

Explanation:

To find this we can use the rule of sines.

It states
`(sinA)/(a)=(sinB)/(b)=(sinC)/(c)`

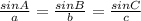
We will use 56 degrees and its complementary measurement, which is discovered by observing the opposite side from the angle, which is 4. Then, we will find the side that compliments x, which is 4.5. Then we can plug those values into the rule of sines.

`(sin56)/(4)=(Sinx)/(4.5)`

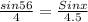
Then, we want to get Sin x by itself.

`(sin56)/(4)*4.5=sinx`

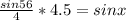
Then, we can solve for sin x.

`0.932667269124=sinx`

finally, we need to take the inverse of sin to find our solution.

`sin^(-1) (0.932667269124)=sin^-^1(sinx)\\x=68.85\\`

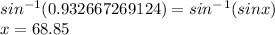
Which can be rounded to 68.9.