Question

A sphere has a volume of 65.5 cubic inches. What is the diameter of the

sphere, to the nearest tenth of an inch?

Answer 1

5 cm

Explanation:

The formula to find the volume of a sphere is:

`\sf V =(4)/(3) \pi r^3`

Here,

V ⇒ volume ⇒ 65.5 cm³

r ⇒ radius

Let us find the value of r.

`\sf V =(4)/(3) \pi r^3\\\\65.5=(4)/(3) \pi r^3\\\\65.5*3=4 \pi r^3\\\\196.5=4 \pi r^3\\\\(196.5)/(4) =\pi r ^3\\\\49.125=\pi r^3\\\\(49.125)/(\pi) = r^3\\\\15.63=r^3\\\\\sqrt[3]{15.63} =r\\\\2.5=r`

Let us find the diameter now.

d = 2r

d = 2 × 2.5

d = 5 cm

Answer 2

5.0 inches

Explanation:

The formula for the volume of a sphere is:

`\boxed{V=(4)/(3)\pi r^3}`

where r is the radius of the sphere.

Given a sphere has a volume of 65.5 cubic inches, substitute V = 65.5 into the formula and solve for the radius, r:

`\begin{aligned}\implies (4)/(3)\pi r^3&=65.5\\\\3 \cdot (4)/(3)\pi r^3&=3 \cdot 65.5\\\\4\pi r^3&=196.5\\\\(4\pi r^3)/(4 \pi)&=(196.5)/(4 \pi)\\\\r^3&=15.636973...\\\\\sqrt[3]{r^3}&=\sqrt[3]{15.636973...}\\\\r&=2.50063840...\; \sf in\end{aligned}`

The diameter of a sphere is twice its radius.

Therefore, if the radius is 2.50063840... inches, then the diameter is:

`\begin{aligned}\implies d&=2r\\&=2 \cdot 2.50063840...\\&=5.00127681...\\&=5.0\; \sf in\;(nearest\;tenth)\end{aligned}`

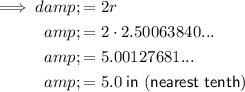
Therefore, the diameter of a sphere with a volume of 65.5 cubic inches is 5.0 inches, to the nearest tenth of an inch.