Question

A dockworker applies a constant horizontal force of 73.0 N to a block of ice on a smooth horizontal floor. The frictional force is negligible. The block starts from rest and moves a distance 13.0 m in a time 4.50 s.

If the worker stops pushing at the end of 4.50 s, how far does the block move in the next 4.20s ? ------ I tried using [distance=0.5at^2] but it says its wrong. how do you do this question?

Answer 1

Approximately
`24.3\; {\rm m}`.

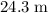Step-by-step explanation:

Under the assumptions, the net force on the block is equal to the horizontal force from the worker.

During the first
`4.50\; {\rm s}` where the worker was applying a constant force on the block, the net force on the block will be constant. Acceleration of the block will be also constant, and SUVAT equations will apply.

Apply the SUVAT equation:

`\displaystyle x &= \left((u + v)/(2)\right)\, t`,

Where:

• 
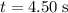  `t = 4.50\; {\rm s}` is the duration of the acceleration,
• 
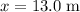  `x = 13.0\; {\rm m}` is the displacement of the block during that
  `4.50\; {\rm s}`,
• 
  `u = 0\; {\rm m\cdot s^(-1)}` is the initial velocity of the block (the block started from rest,) and
• 
  `v` is the velocity of the block after the
  `4.50\; {\rm s}` of acceleration.

(In other words, displacement during constant acceleration is equal to average velocity times the duration of the acceleration.)

Rearrange this equation to find
`v`:

`\begin{aligned}u + v = (2\, x)/(t)\end{aligned}`.

`\begin{aligned}v &= (2\, x)/(t) - u \\ &= (2\, (13.0))/(4.50) - 0 \\ &= (52)/(9)\; {\rm m \cdot s^(-1)}\end{aligned}`.

During the next
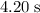`4.20\; {\rm s}`, the net force on the block will be zero. The velocity of the block during that much time will stay unchanged at the final velocity after the initial
`4.50\; {\rm s}`, which is
`v = (52/9)\; {\rm m\cdot s^(-1)}`.

Since velocity during this
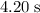`4.20\; {\rm s}` is constant, simply multiply that velocity by the duration to find the distance travelled:

`\displaystyle \left((52)/(9)\right)\, (4.20) \approx 24.3\; {\rm m}`.

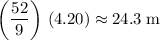In other words, the block would have travelled an additional
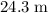`24.3\; {\rm m}` during the
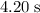`4.20\; {\rm s}`.