Question

The size of fish is very important to commercial fishing. A study conducted in 2012 found the length of Atlantic

cod caught in nets in Karlskrona to have a mean of 49.9 cm and a standard deviation of 3.74 cm (Ovegard, Berndt
& Lunneryd, 2012). Assume the length of fish is normally distributed.

b) What is the length in cm of the longest 15% of Atlantic cod in this area?

Answer 1

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by

`\text{Z}=\frac{\text{X}-\mu}{\sigma}`

After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X.

In this problem, we have that:

A study conducted in 2012 found the length of Atlantic cod caught in nets in Karlskrona to have a mean of 49.9 cm and a standard deviation of 3.74 cm, so
`\mu=49.9,\sigma=3.74`.

What is the length in cm of the longest 15% of Atlantic cod in this area?

We have to find the value of X for the value of Z that has a p-value of 0.85.

Looking at the z-score table, we have that Z = 1.04 has a p-value of 0.8508. So, we have to find the value of X when .

So

`\text{Z}=\frac{\text{X}-\mu}{\sigma}`

`1.04=\frac{\text{X}-49.9}{3.74}`

`\text{X}-49.9=3.8896`

`\text{X}=53.7896`

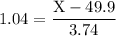
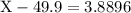
The length of the longest 15% of Atlantic cod in this area is 53.79 cm, rounded to 2 decimal places.