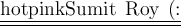The series
 does not converge, because the function
does not converge, because the function
 oscillates infinitely between -1 and 1 as n approaches infinity.
oscillates infinitely between -1 and 1 as n approaches infinity.
More formally, for any positive integer
 , we can find an integer
, we can find an integer
 such that
such that
 is very close to
is very close to
 , which implies that
, which implies that
 . Therefore, the sequence
. Therefore, the sequence
 does not converge to any limit as
does not converge to any limit as
 approaches infinity.
approaches infinity.
Alternatively, we can also prove that
 does not exist using the epsilon-delta definition of a limit. Suppose there exists a limit
does not exist using the epsilon-delta definition of a limit. Suppose there exists a limit
 , then for any
, then for any
 , there exists a positive integer
, there exists a positive integer
 such that for all
such that for all
 , we have
, we have
 .
.
However, we can choose
 and find two subsequences
and find two subsequences
 and
and
 such that
such that
 . Therefore, for any possible limit
. Therefore, for any possible limit
 , we can always find two subsequences that converge to different values, contradicting the assumption that the limit exists.
, we can always find two subsequences that converge to different values, contradicting the assumption that the limit exists.


♥️