Question

Calculate the pressure (in atm) of 0.521 mole of helium gas at 254 K when it occupies a volume of 3.32 L.

Answer 1

3.27 atm (3 s.f.)

Step-by-step explanation:

To calculate the pressure of the helium gas, we can use the Ideal Gas Law.

Ideal Gas Law

`\boxed{PV=nRT}`

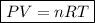
where:

• P is the pressure measured in atmosphere (atm).
• V is the volume measured in liters (L).
• n is the number of moles.
• R is the ideal gas constant (0.082057366080960 atm L mol⁻¹ K⁻¹).
• T is the temperature measured in kelvin (K).

The values to substitute into the equation are:

• V = 3.32 L
• n = 0.521 mol
• R = 0.082057366080960 atm L mol⁻¹ K⁻¹
• T = 254 K

Rearrange the formula to isolate P:

`\implies PV=nRT`

`\implies P=(nRT)/(V)`

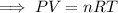
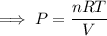
Substitute the values into the formula and solve for P:

`\implies P=\sf (0.521 \;mol \cdot 0.082057 \;atm \;L\;mol^(-1) \;K^(-1) \cdot 254 \;K)/(3.32 \;L)`

`\implies P=\sf (0.521 \cdot 0.082057 \;atm \cdot 254)/(3.32)`

`\implies P=\sf (10.8589794... \;atm)/(3.32)`

`\implies P=\sf 3.27077695...\;atm`

`\implies P=\sf 3.27\;atm\;(3\;s.f.)`

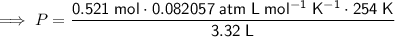
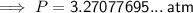
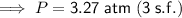
Therefore, the pressure of the helium gas is 3.27 atm (to three significant figures) when it occupies a volume of 3.32 L at 254 K.