Question

F(x)=2x^(2)-3x-5 write in vertex form

Answer 1

`\boxed{f(x)=(√(2)x-(3)/(2√(2) )) ^2-(49)/(8)}`

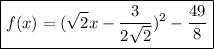
Explanation:

To convert our function
`f(x)=2x^(2)-3x-5` to the vertex form, we must express it in the following form:

`f(x) = a(x - h)^2 + k`

where:

• 
  `a=` vertical compression
• 
  `h=` horizontal traslation
• 
  `k=` vertical translation

To convert the function, I will look for a square binomial that gives me the first two terms of our function.

`\begin{aligned} (√(2)x-(3)/(2√(2) )) ^2& = 2x^2 - (3)/(2)x-(3)/(2)x+(3^2)/(2^2(2))\\ (√(2)x-(3)/(2√(2) )) ^2 &=2x^2-3x +(9)/(8) \\ (√(2)x-(3)/(2√(2) )) ^2-(9)/(8)&=2x^2-3x \end{aligned}`

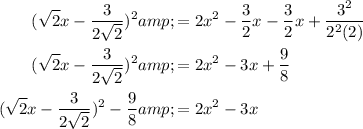
Now, I substitute
`2x^2-3x`

`f(x)=(√(2)x-(3)/(2√(2) )) ^2-(9)/(8)-5\\f(x)=(√(2)x-(3)/(2√(2) )) ^2-(49)/(8)`

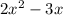
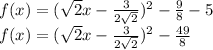
Hope it helps

`\text{-B$\mathfrak{randon}$VN}`