Question

Calculate the pH for each of the following cases in the titration of 35.0 mL of 0.120 M KOH(aq), with 0.120 Number Note: Enter your answers with two decimal places. (a) before addition of any HI Number (b) after addition of 13.5 mL of H Number (c) after addition of 25.5 mL of HI Number (d) after the addition of 35.0 mL of H Number (e) after the addition of 45.5 mL of H Number after the addition of 50.0 mL of H L

Answer 1

Step-by-step explanation:

Before the addition of any HI, the pH of 0.120 M KOH is equal to 14-pOH+, where pOH is the negative log of the concentration of OH+ ions. Since KOH is a strong base and dissociates completely, the concentration of OH+ ions is the same as the concentration of KOH atoms, 0.120 M.

`pOH=-log(0.120)\\pOH=0.921\\\\pH+pOH=14\\14-pOH=pH\\14-0.921=pH\\pH=13.1`

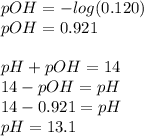
a). pH = 13.1

For the next 4 parts, we will use this equation for the titration of KOH with KI

KOH + HI ⇒ H₂O + KI

or, the net ionic equation

OH⁺ + H⁻ = H₂O

The moles of KOH is equal to the concentration * the volume in liters, so

0.120*0.035 = 0.0042 mol OH+

in part b, we have 13.5 ml of HI with 0.120 concentration, so

0.120*0.0135 = 0.00162 mol H-

In this problem, OH+ is the excess reactant upon completion since there are more moles present of it in a 1:1 ratio of reactants, so we will use the excess OH+ ions to determine pOH and then pH.

H- is the limiting reactant, so 0.00162 moles of H- and OH+ will be used, leaving 0.0042-0.0026 moles of OH+ left. The concentration is then this number over the total volume: 0.035 L KOH + 0.0135 L HI, which is a total of 0.0485 L.

Thus, the concentration of OH+ is
`(0.0026)/(0.0485) = 0.053 M`

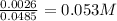
The pOH is then -log(0.053) = 1.27

pH = 14 - pOH = 12.73. This answer is lower than that for part a, which makes sense as some acid was added which would lower the pH.

apply this same process for parts c,d,e using the new volumes of HI provided to get the pH for each. If you would like me to explain these in depth as well, leave a comment.