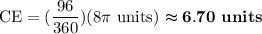Question

In circle D with m/CDE = 96 and CD = 4 units, find the length of arc

CE. Round to the nearest hundredth.

Answer 1

`\text{ CE} \thickapprox 6.70 \ \text{units}`

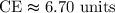
Explanation:

The circumference of a circle is given by the formula ...

`\text{C} = 2\pi r`

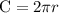
Your circle has a radius CD of 4 units, so its circumference is ...

`\text{C} = 2\pi (4 \ \text{units}) = 8\pi \ \text{units}`

__

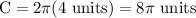
The arc of interest is 96°. The whole circle is 360°, so the length of the arc is 96/360 times the circumference of the circle:

`\text{CE} = ((96)/(360) )(8\pi \ \text{units}) \bold{\ \thickapprox 6.70 \ units}`