Question

A monkey slips on a banana peel and slides off the edge of a short, flat cliff and fell into the

pond below. the monkey slides off the edge with velocity of 2.0 m/s and the cliff is 1.5 meters
above the water level

How long does it take the monkey to hit the pond?

How far from the base of the cliff does the monkey hit the pond?

Answer 1

The monkey hit the pond after approximately
`0.55\; {\rm s}` in the air.

The monkey hit the pond approximately
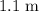`1.1\; {\rm m}` away from the base of the cliff.

(Assume that
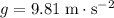`g = 9.81\; {\rm m\cdot s^(-2)}`, air resistance on the monkey is negligible, and the cliff is vertical.)

Step-by-step explanation:

Assume that the air resistance on the monkey is negligible. The vertical acceleration of the monkey would be constantly
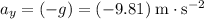`a_(y) = (-g) = (-9.81)\; {\rm m\cdot s^(-2)}`. Note that
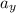`a_(y)` is negative since the monkey is accelerating downwards.

Right before hitting the pond, the monkey would be
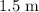`1.5\; {\rm m}` below the cliff. Hence, the vertical displacement
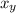`x_(y)` of the monkey would be
`(-1.5)\; {\rm m}`.

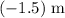Let
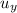`u_(y)` denote the initial vertical velocity of the monkey. Since the top of the cliff is level, initial velocity will be entirely horizontal. Hence,
`u_(y) = 0\; {\rm m\cdot s^(-1)}`.

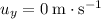Let
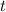`t` denote the amount of time it took for the monkey to hit the pond (
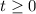`t \ge 0`.) Rearrange the SUVAT equation
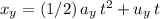`x_(y) = (1/2)\, a_(y)\, t^(2) + u_(y)\, t` and solve for
`t\!`.

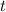Since
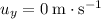`u_(y) = 0\; {\rm m\cdot s^(-1)}`, the equation simplifies to:

`x_(y) = (1/2)\, a_(y)\, t^(2)`.

`\begin{aligned}t^(2) = (2\, x_(y))/(a_(y))\end{aligned}`.

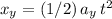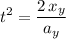Since
`t \ge 0`:

`\begin{aligned}t &= \sqrt{(2\, x_(y))/(a_(y))} \\ &= \sqrt{\frac{(2)\, (1.5\; {\rm m})}{(9.81\; {\rm m\cdot s^(-2)})}} \\ &\approx 0.553\; {\rm s}\end{aligned}`.

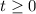Hence, it would take approximately
`0.55\; {\rm s}` for the monkey to hit the pond.

Also because air resistance on the monkey is negligible, the horizontal velocity
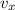`v_(x)` of the monkey will be constantly equal to the initial value
`2.0\; {\rm m\cdot s^(-1)}`.

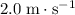Hence, the monkey would have travelled a horizontal distance
`x_(x)` of:

`\begin{aligned} x_(x) &= v_(x)\, t \\ &\approx (2.0\; {\rm m\cdot s^(-1)})\, (0.553\; {\rm s})\\ &\approx 1.1\; {\rm m}\end{aligned}`.