Question

Please help and explain

Answer 1

well, first off, hmmm let's find their slopes in the first place

`p=\stackrel{\stackrel{m}{\downarrow }}{2}n+5\qquad \impliedby \qquad \begin{array} \cline{1-1} slope-intercept~form\\ \cline{1-1} \\ y=\underset{y-intercept}{\stackrel{slope\qquad }{\stackrel{\downarrow }{m}x+\underset{\uparrow }{b}}} \\\\ \cline{1-1} \end{array}\qquad {\Large \begin{array}{llll} \boxed{x=2} \end{array}}`

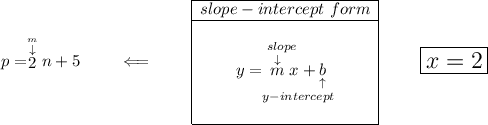
now, to get the equation of any straight line, we simply need two points off of it, let's use those two in the picture below for BrandY
`(\stackrel{x_1}{1}~,~\stackrel{y_1}{3})\qquad (\stackrel{x_2}{7}~,~\stackrel{y_2}{9}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{\textit{\large rise}} {\stackrel{y_2}{9}-\stackrel{y1}{3}}}{\underset{\textit{\large run}} {\underset{x_2}{7}-\underset{x_1}{1}}} \implies \cfrac{ 6 }{ 6 } \implies {\Large \begin{array}{llll} 1=Y \end{array}}`

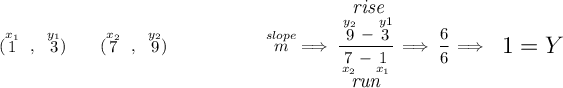
to get the equation of any straight line, we simply need two points off of it, let's use those two in the picture above for BrandZ

`(\stackrel{x_1}{0}~,~\stackrel{y_1}{3})\qquad (\stackrel{x_2}{4}~,~\stackrel{y_2}{9}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{\textit{\large rise}} {\stackrel{y_2}{9}-\stackrel{y1}{3}}}{\underset{\textit{\large run}} {\underset{x_2}{4}-\underset{x_1}{0}}} \implies \cfrac{ 6 }{ 4 } \implies {\Large \begin{array}{llll} \cfrac{3 }{ 2 }=Z \end{array}} \\\\[-0.35em] ~\dotfill\\\\ ~\hfill \stackrel{X}{2} ~~ > ~~ \stackrel{Y}{1}\hspace{5em}\stackrel{X}{2}~~ > ~~ \stackrel{Z}{(3)/(2)}~\hfill`