Question

What should be added to 9x2-12xy to make it a perfect square

Answer 1

4y^2

Explanation:

`9 {x}^(2) - 12xy`

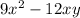
9 is a square of 3

Let's write a formula for the square of the difference:

`{(x - y)}^(2) = {x}^(2) - 2xy + {y}^(2)`

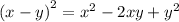
In the first place we can write 3x, because:

`(3 {x})^(2) = 9 {x}^(2)`

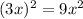
Now, -12xy could be the product of 3x and -4y (but it has to be half the product, so we write -2y instead)

In order to get a perfect square, we need to choose such numbers that can be changed in the form of this formula:

(3x - 2y)^2 = 9x^2 - 12xy + 4y^2

That means, 4y^2 must be added

Answer 2

Explanation:

a perfect square is

(a + b)²

as in our case this involves x and y, we can outright write

(ax + by)²

now, let's do the multiplication :

a²x² + 2abxy + b²y²

what do we have ?

9x²

that fits to a²x² and means that a = 3.

-12xy

that must be 2abxy. we know, a = 3.

-12xy = 2×3×bxy

-2xy = bxy

so, b = -2

that means what is missing is b²y² = 4y²

the perfect square would then be

9x² - 12xy + 4y²