Question

Learning Task 3 : Solve the problem. Provide an illustration if necessary. ( 3 points each)

1.The length of o a rectangle is 12 cm and its width is 2 cm less than ¾ of its length. Find the
area of a rectangle .








2.A circular clock with a circumference of 88 cm, is mounted on the wall. How much area of
the wall did it occupy ( Use : π = 22/7 ).




3. The length of a rectangle is 52 cm and its perimeter is 200 cm . What is the area of the rectangle?

Answer 1

Explanation:

1. 84 cm^2

2. 616 cm^2

3. 2496 cm^2

Given:

A triangle

l (length) = 12 cm

w (width) is 2 cm less than 3/4 of its length

Find: A (area) - ?

First, let's find the width of the rectangle according to the given information:

`w = ((3)/(4) * 12) - 2 = 9 - 2 = 7 \: cm`

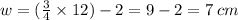
Now, we can find the area:

`a = w * l`

.

2. Given:

A circular clock

C (circumference) = 88 cm

π = 22/7

Find: A (area) - ?

`c = 2\pi * r`

First, let's find the radius of the clock:

`2 * (22)/(7) * r = 88`

`(44)/(7) * r = 88`

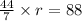
Multiply both sides of the equation by 7 to eliminate the fraction:

`44r =616`

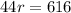
Divide both parts of the equation by 44 to make r the subject:

`r = 14`

Now, we can find the area:

`a = \pi {r}^(2)`

`a = (22)/(7) * {14}^(2) = 616 \: {cm}^(2)`

.

3. Given:

A rectangle

l (length) = 52 cm

P = 200 cm

Find: A (area) - ?

First, let's find the width of the rectangle (the perimeter is equal to the sum of all side lengths):

P = 2l + 2w

2w = P - 2l

2w = 200 - 2 × 52

2w = 96 / : 2

w = 48 cm

Now, we can find the area:

A = w × l

A = 48 × 52 = 2496 cm^2