Question

The specific heat of marble is 0.858 J / g How much heat (in J) is required to raise the temperature of 20.0 g of marble from 22 °C to 45 °C?

Answer 1

`\blue{\huge {\mathrm{SPECIFIC \; HEAT \; CAPACITY}}}`

`\\`

`{===========================================}`

`{\underline{\huge \mathbb{Q} {\large \mathrm {UESTION : }}}}`

• The specific heat of marble is 0.858 J/g. How much heat (in J) is required to raise the temperature of 20.0 g of marble from 22°C to 45°C?

`{===========================================}`

`{\underline{\huge \mathbb{A} {\large \mathrm {NSWER : }}}}`

• The amount of heat required to raise the temperature of 20.0 g of marble from 22°C to 45°C is 394.68 Joules.

`{===========================================}`

`{\underline{\huge \mathbb{S} {\large \mathrm {OLUTION : }}}}`

The formula for calculating the amount of heat required to raise the temperature of an object is:

• 
  `\sf Q = m \cdot c \cdot \Delta T`

where

• Q is the amount of heat required (in Joules),
• m is the mass of the object (in grams),
• c is the specific heat of the object (in Joules per gram degree Celsius), and
• 
  `\bold{\Delta T}` is the change in temperature (in degrees Celsius).

Using the given values, we can plug them into the formula:

`\begin{aligned}\sf Q& =\sf 20.0\: g \cdot 0.858\: J/g^(\circ)C \cdot (45^(\circ)C - 22^(\circ)C)\\& =\sf 20.0\: g \cdot 0.858\: J/g^(\circ)C \cdot 23^(\circ)C \\& = \boxed{\bold{394.68\: J}}\end{aligned}`

Therefore, the amount of heat required to raise the temperature of 20.0 g of marble from 22°C to 45°C is 394.68 Joules.

`{===========================================}`

`- \large\sf\copyright \: \large\tt{AriesLaveau}\large\qquad\qquad\qquad\qquad\qquad\qquad\qquad\tt 04/02/2023`

Answer 2

394.68 J

Step-by-step explanation:

The amount of heat gained or lost by an object when its temperature changes can be calculated by using the formula:

Specific Heat Capacity

`\boxed{\sf c = (Q)/(m \cdot \Delta T)}`

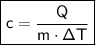
where:

• c is the specific heat of the object.
• Q is the heat gained or lost in joules (J).
• m is the mass of the object.
• ΔT is the change in temperature.

The initial temperature of the marble was 22°C and its final temperature is 45°C. Therefore, the change in temperature, ΔT, is:

`\implies \sf \Delta T=45^(\circ)C-22^(\circ)C=23^(\circ)C`

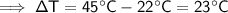
Therefore, the values to substitute into the formula are:

• m = 20.0 g
• c = 0.858 J / (g · °C)
• ΔT = (45°C - 22°C) = 23°C

Substitute these values into the formula:

`\implies \sf \frac{0.858\;J}{g \cdot \!\!\!\!\phantom{2}^(\circ)C}}=(Q)/(20.0\;g \cdot 23 ^(\circ)C)`

`\implies \sf Q=\frac{0.858\;J \cdot 20.0\;g \cdot 23^(\circ)C}{g \cdot \!\!\!\!\phantom{2}^(\circ)C}}`

`\implies \sf Q=0.858\;J \cdot 20.0 \cdot 23`

`\implies \sf Q=394.68\;J`

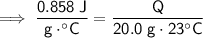
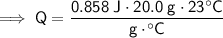
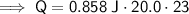
Therefore, 394.68 J of heat is required to raise the temperature of 20.0 g of marble from 22°C to 45°C.