Question

In a survey of 2954 adults, 1401 say they have started paying bills online in the last year.

Construct a 99% confidence interval for the population proportion. Interpret the results.
A 99% confidence interval for the population proportion is
(Round to three decimal places as needed.)
Pls help

Answer 1

The 99% confidence interval for the population proportion is between (0.4526, 0.4960). The interpretation is that we are 99% sure that the true proportion of adults who have started paying bills online in the last year is between these two values.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi (1-\pi ))/(n) }`

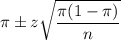
In which

z is the z-score that has a p-value of
`1-(\alpha )/(2)`.

For this problem, we have that:

In a survey of 2954 ​adults, 1401 say they have started paying bills online in the last year. This means that
`n=2954, \ p=(1401)/(2954) =0.4743`

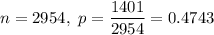
99% confidence level

So
`\alpha =0.01`, z is the value of Z that has a p-value of
`1-(0.01)/(2) =0.995`, so
`Z=2.575`.

The lower limit of this interval is:

`\pi -z\sqrt{(\pi (1-\pi))/(n) } =0.4743-2.575\sqrt{(0.4743*0.4408)/(2954) } =0.4526`

The upper limit of this interval is:

`\pi -z\sqrt{(\pi (1-\pi))/(n) } =0.4743+2.575\sqrt{(0.4743*0.4408)/(2954) } =0.4960`

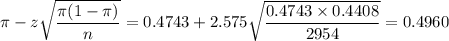
The 99% confidence interval for the population proportion is between (0.4526, 0.4960). The interpretation is that we are 99% sure that the true proportion of adults who have started paying bills online in the last year is between these two values.