Question

Nitrogen gas at 298 K and

75.5 kPa increases in pressure
to 175 kPa.

What is the temperature of the
gas at 175 kPa?

[?] K

Answer 1

Gay-Lussac's Law-

`\:\:\:\:\:\:\star\longrightarrow \underline{\sf \boxed{\sf (P_1)/(T_1)=(P_2)/(T_2)}}`

`\:\:\:\:\:\:\star\longrightarrow \sf \underline{T_2=(T_1 \:P_2)/(P_1)}`

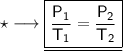
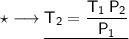
Where-

• P₁ is the initial pressure.
• T₁ is the initial temperature
• P₂ is the final pressure.
• T₂ is the final temperature

As per question, we are given -

• P₁ = 75.5 KPa
• T₁ =298 K
• P₂= 175KPa

Now that we are given all the required values, so we can put them into the formula and solve for T₂:-

`\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\star\longrightarrow \sf \underline{T_2=(T_1 \:P_2)/(P_1)}`

`\:\:\:\:\:\:\:\:\:\: \:\:\:\:\:\:\longrightarrow \sf T_2=(298 * 175)/(75.5)`

`\:\:\:\:\: \:\:\:\:\:\:\:\:\:\:\:\longrightarrow \sf T_2=(52150)/(75.5)`

`\:\:\:\:\:\:\:\:\:\: \:\:\:\:\:\:\longrightarrow \sf T_2=690.728476......`

`\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\longrightarrow \sf T_2=690.73 \:K`

`\:\:\:\:\:\:\:\:\:\: \:\:\:\:\:\:\longrightarrow \sf T_2=(690.73-273)°C`

`\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\longrightarrow \sf \underline{T_2=417.73\:°C}`

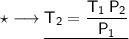
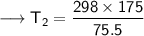
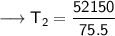
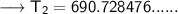
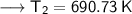
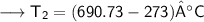
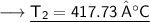
Therefore, the temperature of the gas at 175 kPa will become 690.73 K or, 417.73°C.