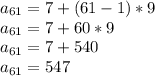Question

Find the 61st term of the following arithmetic sequence7,16,25,34

Answer 1

61st term = 547

Explanation:

The formula for the nth term of an arithmetic sequence is

`a_(n)=a_(1)+(n-1)d`, where a1 is the first term, n is the term position (e.g., 1st or 61st) and d is the common difference.

We already know that a1 = 7 and the n = 61. We can find the common difference by finding the difference of any two consecutive terms with the smaller term being subtracting from the larger:

Thus, if we use 7 and 16, our common difference (d) is:

d = 16 - 7 = 9

Now, we can simply plug in everything to the formula to find the 61st term:

`a_(61)=7+(61-1)*9\\ a_(61)=7+60*9\\ a_(61)=7+540\\ a_(61)=547`