Question

The length of one of the legs of a right triangle is 13. The lengths of the other two sides are consecutive integers. Use the Pythagorean theorem to solve for the the larger of the two missing sides (the hypotenuse).

Answer 1

Leg 1 = 13

Leg 2 = 84

Hypotenuse = 85

Explanation:

Hello!

The larger of the other two legs would be the hypotenuse, as the hypotenuse is the largest side in a right triangle (as stated in the problem.

The Pythagorean theorem states that the sum of the squares of the legs is equal to the square of the hypotenuse.

Let the smallest of the other legs be x, and the hypotenuse be x + 1.

The equation is:
`13^2 + x^2 = (x+1)^2`

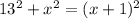
Solve for x:

• 
  `13^2 + x^2 = (x+1)^2`
• 
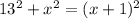
  `169 + x^2 = x^2 + 2x + 1` => Expand
• 
  `169 = 2x + 1` => Subtract x² from both sides
• 
  `168 = 2x` => Subtract 1 from both sides
• 
  `x = 84` => Divide both sides by 2

We found the value for x, so we can find the hypotenuse by adding 1, which gives us 85.