Question

Let r = (x^2 + y^2)^1/2 and consider the vector field F = r^A(-yi + xj), where r 0 and A is a constant. F has no z-component and is independent of z. (a) Find curl r^A(-yi + xj)), and show that it can be written in the form curl F = _____ r^a k, where α = ____, for any constant A. (b) Using your answer to part (a), find the direction of the curl of the vector fields with each of the following values of.4 (enter your answer as a unit vector in the direction of the curl): A = -5 direction = ______

A = 2 direction = _____ (c) For each values of.4 in part (b), what (if anything) does your answer to part (b) tell you about the sign of the circulation around a small circle oriented counterclockwise when viewed from above, and centered at (1, 1, 1)? If A = -5, the circulation is ______ If A = 2, the circulation is ______

Answer 1

To find the curl of the vector field, we can calculate the cross product of the gradient operator and the given vector field. The curl of F is given by curl F = (1/r)(d(rFy)/dx - d(rFx)/dy) k, where r = x²+ y²) ¹²and F = rA(-yi + xj).

Step-by-step explanation:

To find the curl of the vector field, we can calculate the cross product of the gradient operator and the given vector field. The curl of F is given by curl F = (1/r)(d(rFy)/dx - d(rFx)/dy) k, where r = (x² + y²)1/2 and F = rA(-yi + xj). To simplify the expression, we substitute Fy = -x² and Fx = y². Then, we differentiate these expressions with respect to x and y and determine the curl in terms of A. The result is curl F = -2A(k + αr), where α = 1/r.

Answer 2

(a) The curl of the vector field
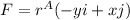`\( F = r^A(-yi + xj) \)` is {Curl} F =
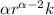`\alpha r^(\alpha-2)k \)`, where
`\( \alpha = A - 1 \).`

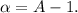
(b) For ( A = -5 ), the direction of the curl is ( k ); for ( A = 2 ), the direction is ( -k ).

(c) If ( A = -5 ), the circulation around a small counterclockwise-oriented circle centered at (1, 1, 1) is positive; if ( A = 2 ), the circulation is negative.

Step-by-step explanation:

(a) To find the curl of F =
`r^A`(-yi + xj), we can use the expression for the curl in cylindrical coordinates. The curl turns out to be αr^(α-2)k, where α is the constant A - 1.

(b) To determine the direction of the curl, we look at the k-component of the curl. For A = -5, the direction is given by k; for A = 2, the direction is -k.

(c) The sign of the curl's k-component indicates the circulation direction when viewed from above. For A = -5, the counterclockwise direction is consistent with a positive k-component. For A = 2, the clockwise direction corresponds to a negative k-component. Thus, the answers in part (b) suggest that the circulation around a small counterclockwise-oriented circle centered at (1, 1, 1) is positive when A = -5 and negative when A = 2.

In conclusion, the curl of the vector field F is determined by the constant α = A - 1, and its k-component provides information about the circulation direction. The results indicate that the sign of the circulation depends on the value of A, with counterclockwise circulation for A = -5 and clockwise circulation for A = 2.