Question

You need to solve a system of equations. You decide to use the elimination method. Which of these is not allowed? 2x-3y=12 Equation 1 -2x+y=8 Equation 2 A. Add equation 2 to equation 1. B. Multiply equation 2 by 3. Then subtract the result from equation 1. C. Add the left side of equation 2 to the left side of equation 1.

Answer 1

Answer: Choice B.

Multiply equation 2 by 3. Then subtract the result from equation 1.

Step-by-step explanation:

The 2nd equation given to us is -2x+y = 8

Triple both sides to get -6x+3y = 24

This means the original system

`\begin{cases}2x-3y = 12\\-2x+y = 8\end{cases}`

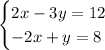
is equivalent to

`\begin{cases}2x-3y = 12\\-6x+3y = 24\end{cases}`

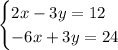
If we were to add the equations, then the y terms would cancel out because -3y+3y = 0y = 0.

Subtracting the equations will not cancel out the y terms.

-3y minus 3y = -3y -3y = -6y

Therefore the portion saying "Then subtract the result from equation 1." in answer choice B is not correct. It should be "add the equations" or "add the result to equation 1" after tripling both sides of the 2nd equation.

Answer choices A and C say the same thing more or less. Adding the original equations straight down will cancel out the x terms. Therefore, choices A and C are valid approaches to using elimination. We can cross choices A and C off the list.