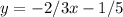Answer:
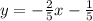
Explanation:
Given
Passes through:
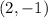
Parallel to:
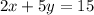
Required
First, calculate the slope of the parallel line
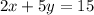
Make y the subject
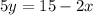
Divide through by 5
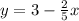
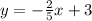
An equation in slope intercept has the form:

Where:
m = slope

So:
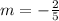
The required is parallel to
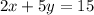 .
.
This mean, the same slope
The equation is the calculated using:
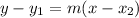
This gives:
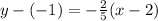
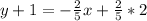

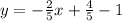
Take LCM
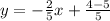
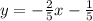
i.e.