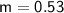Question

If the change in internal

energy = 1943J, specific heat
capacity = 90J/°C/kg, and temperature change = 41°C, what is the mass of the object?
Give your answer to 2 decimal places. Help

Answer 1

The mass of the object is 0.53 kg.

Step-by-step explanation:

We can use the internal energy of an ideal gas equation do evaluate the mass.

`\sf \Delta U=mc \Delta T`

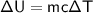
Where

`\sf \Delta U` is the change in internal energy

`\sf m` is the mass of the object

`\sf c` is the specific heat capacity of the object

`\sf \Delta T` is the change in temperature

We can rearrange the equation to isolate the mass (
`\sf m`).

Divide both sides of the equation by
`\sf c \Delta T`.

`\sf (\Delta U)/(\sf c \Delta T) = \sf (mc \Delta T)/(\sf c \Delta T)`

`\sf c \Delta T` cancels out on the right side leaving us with

`\sf (\Delta U)/(\sf c \Delta T) = \sf m`

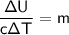
Numerical Evaluation

In this example we are given

`\sf \Delta U=1943` J

`\sf c=90` J/°C/kg

`\sf \Delta T=41` °C

Substituting our given values into the equation yields

`\sf m=\sf (1943)/(90 \cdot 41)`

`\sf m=0.526558265`

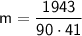
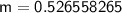
Rounding to 2 decimal places leaves us with

`\sf m=0.53`