Question

Which is the equation of a parabola with a directrix at y = 2 and a focus at (5, 0).

y = one fourth(x − 5)2 + 1
y = one fourth(x + 1)2 − 5
y = −one fourth(x + 1)2 − 5
y = −one fourth(x − 5)2 + 1

Answer 1

y = -
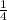`(1)/(4)` (x - 5)² + 1

Explanation:

any point (x, y) on a parabola is equidistant from the directrix and the focus.

using the distance formula

`√((x-5)^2+(y-0)^2)` = | y - 2 | ← square both sides

(x - 5)² + y² = (y - 2)² = y² - 4y + 4 ( subtract y² from both sides )

(x - 5)² = - 4y + 4 ( add 4y to both sides )

4y + (x - 5)² = 4 ← subtract (x - 5)² from both sides

4y = - (x - 5)² + 4 ← divide through by 4

y = -
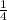`(1)/(4)` (x - 5)² + 1