Question

Karen spent $52 on 5 buns and 7 cakes . A cake cost thrice as much as a bun. Find the cost of 2 cakes​

Answer 1

$12

Explanation:

Let's assume that buns cost x and cakes y dollars

Now, let's write two equations according to the given information and put them in a system:

`5x + 7y = 52`

`(y)/(x) = 3`

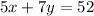
From the 2nd equation: y = 3x

Replace y in the 1st equation:

`5x + 7 * 3x = 52`

`5x + 21x = 52`

`26x = 52`

`x = 2`

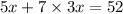
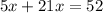
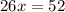
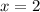
We found the price of one bun

Since we know that one cake costs thrice as much as a bun, we can find the price of one bun:

y = 3 × 2 = 6

Now multiply this number by 2 and we'll get the answer

2 × 6 = $12

Answer 2

Cost Of 2 Cakes = $12

━━━━━━━━━━━━━━━━━━━━━━

Let ::

Cost Of Bun = $x

Cost Of Cake = $3x

ㅤ

Then ::

Cost Of 5 Buns = $5x

Cost Of 7 Cakes = $(7 × 3x) = $21x

ㅤ

Atq ::

`\begin{gathered} \\ \; \; \sf{:\longmapsto{5x + 21x = 52}} \\ \\ \end{gathered}`

`\begin{gathered} \\ \; \; \sf{:\longmapsto{26x = 52}} \\ \\ \end{gathered}`

`\begin{gathered} \\ \; \; \sf{:\longmapsto{x = \cancel{(52)/(26)}}} \\ \\ \end{gathered}`

`\begin{gathered} \\ \; \; :\longmapsto{\underline{\boxed{\orange{\frak{x = 2}}}}} \; \pmb{\bigstar} \\ \\ \end{gathered}`

• Substituting The Value Of (x)

ㅤ

➔ Cost Of Bun = $2

➔ Cost Of Cake = $(3 × 2) = $6

ㅤ

Now ::

`\begin{gathered} \\ \; \; \sf{Cost \; Of \; 2 \; Cakes:-} \\ \\ \end{gathered}`

`\begin{gathered} \\ \; \; \sf{:\longmapsto{\${(6 * 2)}}} \\ \\ \end{gathered}`

`\begin{gathered} \\ \; \; :\longmapsto{\underline{\boxed{\frak{ \: \: \$ \: {12} \: \: }}}} \; \pmb{\red{\bigstar}} \\ \\ \end{gathered}`

`\bf{\pmb{\underline{\rule{170pt}{5pt}}}}`