Answer:
Approximately
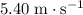 (assuming that
(assuming that
 .)
.)
Step-by-step explanation:
Refer to the diagram attached. There are two forces on this tetherball: tension
 in the string, and weight
in the string, and weight
 - where
- where
 is the mass of the tetherball.
is the mass of the tetherball.
Let
 denote the tension in the string, and let
denote the tension in the string, and let
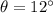 denote the angle of elevation of this force. Decompose
denote the angle of elevation of this force. Decompose
 into two components: horizontal and vertical:
into two components: horizontal and vertical:
- Vertical:
 , and
, and - Horizontal:
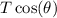 .
.
The tetherball is moving in a horizontal plane, meaning that there is no motion in the vertical direction. Hence, the resultant force in the vertical direction should be
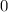 . The vertical component of the tension
. The vertical component of the tension
 should exactly balance the weight of the tetherball
should exactly balance the weight of the tetherball
 :
:
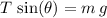 .
.
Hence, the resultant (unbalanced) force on this tetherball would be equal to the horizontal component of tension:
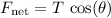 .
.
The length of the rope is
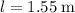 . Since this rope is also at the angle of
. Since this rope is also at the angle of
 above the horizon, the radius of the circular motion in the horizontal plane would be
above the horizon, the radius of the circular motion in the horizontal plane would be
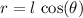 .
.
Since the ball is in a centripetal motion, the resultant force on this ball would also be
 , where
, where
 is the velocity of the ball.
is the velocity of the ball.
Equate these two expressions of
 to obtain:
to obtain:
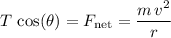 .
.
Additionally,
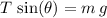 since the forces on the vertical direction are balanced. Rewrite both this equation and the equation
since the forces on the vertical direction are balanced. Rewrite both this equation and the equation
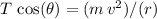 to isolate tension
to isolate tension
 :
:
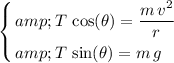 .
.
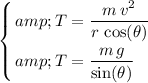 .
.
Solve this system for velocity
 :
:
 .
.
Since
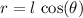 :
:
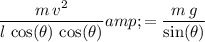 .
.
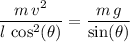 .
.
 .
.