Question

Graph the inequality.
x-7y≤0
I need the points that goes on the graph

Answer 1

first off hmmm let's solve for "y"

`x-7y\leqslant 0\implies -7y\leqslant -x\implies y\stackrel{ \stackrel{notice}{\downarrow } }{\geqslant} \cfrac{-x}{-7}\implies y\geqslant \cfrac{x}{7}`

first off, we have "⩾", that means our inequality includes all values greater than and also all values on the border line, so since the values of the borderline are included, that means our line is going to be a solid line.

Now, before we graph the inequality, let's first off get the graph of the equivalent "equality", that is, the equation of y = x/7. So hmmm we can pick any two random "x" values to graph that, now, once that's graphed, where the heck do we shade?

well, to know which part to shaded, we do a true/false check on the regions, we only need to know one of the regions really, so since it's a line it only has two regions, if one is false, the other must be the true one, so hmm to do that we pick a point hmm say (2 , 1), that point is above our solid line, now let's check if that's true

`y\geqslant \cfrac{x}{7}\hspace{5em}(\stackrel{x}{2}~~,~~\stackrel{y}{1})\hspace{5em}1\geqslant \cfrac{2}{7}\qquad \impliedby \textit{is that really \underline{true}?} \\\\\\ \textit{well, is true, because }1\textit{ is indeed greater than }\cfrac{2}{7}`

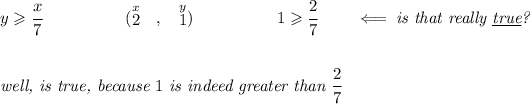
that means that the point (2 , 1) is on the true region, so that's the region that gets shaded. Check the picture below.

Answer 2

x - 7y
`\leq` 0 = y
`\geq` 1/7x