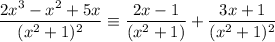Question

PLEASE HELP!!

Decomposing a Fraction with a Repeated Irreducible Quadratic Factor
Find the partial fraction decomposition of 2x^ 3 -x^ 2 +5x (x^ 2 +1)^ 2
Please show work

Answer 1

`(2x^3-x^2+5x)/((x^2+1)^2)\equiv (2x-1)/((x^2+1))+(3x+1)/((x^2+1)^2)`

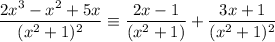
Explanation:

As the denominator has a repeated irreducible quadratic factor, and the degree of the denominator is greater than the degree of the numerator, the partial fraction form is:

`\boxed{(N(x))/((x^2+c)^2) \equiv(Ax+B)/((x^2+c))+(Cx+D)/((x^2+c)^2)}`

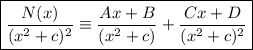
Therefore, the given algebraic fraction can be written as partial fractions of the form:

`(2x^3-x^2+5x)/((x^2+1)^2)\equiv (Ax+B)/((x^2+1))+(Cx+D)/((x^2+1)^2)`

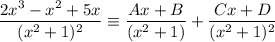
Add the partial fractions:

`(2x^3-x^2+5x)/((x^2+1)^2)\equiv ((Ax+B)(x^2+1)+Cx+D)/((x^2+1)^2)`

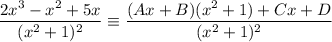
Cancel the denominators from both sides of the original identity, so the numerators are equal:

`2x^3-x^2+5x = (Ax+B)(x^2+1)+Cx+D`

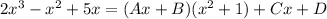
Expand the right side of the equation:

`2x^3-x^2+5x=Ax^3+Ax+Bx^2+B+Cx+D`

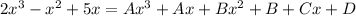
Group elements according to the powers of x:

`2x^3-x^2+5x=Ax^3+Bx^2+(A+C)x+B+D`

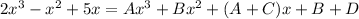
Equate the coefficients of the terms in x³ and x² to solve for A and B:

`\implies A=2`

`\implies B=-1`

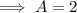
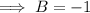
Substitute the found values of A and B into the equation:

`2x^3-x^2+5x=2x^3-x^2+(2+C)x-1+D`

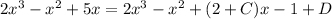
Equate the coefficients of the terms in x and the constant to solve for C and D:

`\implies 5=2+C \implies C=3`

`\implies 0=-1+D \implies D=1`

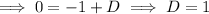
Replace A, B, C and D in the original identity:

`(2x^3-x^2+5x)/((x^2+1)^2)\equiv (2x-1)/((x^2+1))+(3x+1)/((x^2+1)^2)`