Question

Lynn forms a solid by combining a cone and a cylinder.

What is the volume, in cubic centimeters, of the solid? Use 3.14 for π. Round your answer to the nearest whole number.

A: 236
B: 311
C: 349
D: 942

Thank you for helping!

Answer 1

A) 236 cm³

Explanation:

To calculate the volume of the composite solid, sum the volume of the cone and the volume of the cylinder.

`\boxed{\begin{minipage}{4 cm}\underline{Volume of a cone}\\\\$V=(1)/(3) \pi r^2 h$\\\\where:\\ \phantom{ww}$\bullet$ $r$ is the radius. \\ \phantom{ww}$\bullet$ $h$ is the height.\\\end{minipage}}`
`\boxed{\begin{minipage}{4 cm}\underline{Volume of a cylinder}\\\\$V=\vphantom{\frac43}\pi r^2 h$\\\\where:\\ \phantom{ww}$\bullet$ $r$ is the radius. \\ \phantom{ww}$\bullet$ $h$ is the height.\\\end{minipage}}`

From inspection of the given diagram, the diameter of the circular base of the cone and cylinder is 6 cm. As the radius is half the diameter, the radius is:

`\implies r=(6)/(2)=3\; \sf cm`

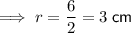
The height of the cone is 4 cm. The height of the composite solid is 11 cm. Therefore, the height of the cylinder is:

`\implies h_(\sf cylinder)=11-4=7\; \sf cm`

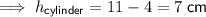
Using π = 3.14, the volume of the composite solid is:

`\begin{aligned}\implies V_(\sf composite\;solid)&=V_(\sf cone)+V_(\sf cylinder)\\\\&=(1)/(3) \cdot 3.14 \cdot 3^2 \cdot 4+3.14 \cdot 3^2\cdot 7\\\\&=(1)/(3) \cdot 3.14 \cdot 9 \cdot 4+3.14 \cdot 9\cdot 7\\\\&=37.68+197.82\\\\&=235.5\\\\&\approx 236\; \sf cm^3\;(nearest\;whole\;number)\end{aligned}`

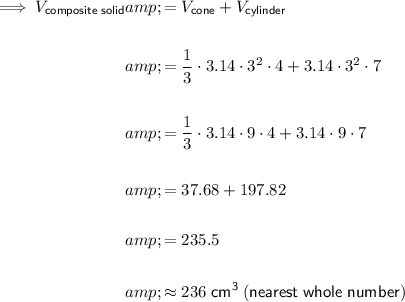
Therefore, the volume of the composite solid is 236 cubic centimeters, rounded to the nearest whole number.