Question

Circle O has a center at (3,1) and a diameter of 10 units. Which point
lies on circle O?

Answer 1

The point (3,-4) will lie on the circle whose centre is at (3,1) and has a diameter of 10 units.

Explanation:

To find which point lies on circle O, we need to use the equation of a circle, which is:

`( x - h )^(2) + ( y - k )^(2) = r^(2)`

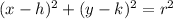
Where (h, k) is the center of the circle and r is the radius. In this case, the center of the circle is at (3,1) and the diameter is 10 units, also the radius is half of the diameter, which is 5 units here.Therefore, the equation of circle

O will be:

`(x - 3 )^(2) + (y+1)^(2) = 5^(2)`

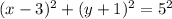
Now to find which point lies on the circle, we need to substitute values for x and y and check for what values does this equation satisfies. On substituting (3,-4) as x and y respectively, we see that:

`(3-3)^(2) + (-4 - 1)^(2) = 25`

`0 + (-5)^(2) = 25`

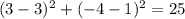
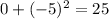
Since the left side of the equation is equal to the right side, the point (3,-4) does lie on the circle O.

Thus the answer to your problem is, The point (3,-4) will lie on the circle whose centre is at (3,1) and has a diameter of 10 units.