Question

gas is escaping a spherical balloon at the rate of 5 \text{ cm}^3 per minute. what is the rate of change of the surface area when the radius is 12 \text{ cm}? please enter your answer in decimal format with three significant digits after the decimal point.

Answer 1

The rate of change of the surface area when the radius is 12 cm is approximately
`\(0.833 \, \text{cm}^2/\text{min}\).`

To find the rate of change of the surface area of a spherical balloon, we can use the formula for the surface area of a sphere:

`\[ A = 4 \pi r^2 \]`

where
`\( A \)` is the surface area and
`\( r \)` is the radius. To find the rate of change
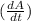`(\( (dA)/(dt) \))` with respect to time, we take the derivative of both sides of the equation:

`\[ (dA)/(dt) = 8 \pi r (dr)/(dt) \]`

Given that the gas is escaping the balloon at a rate of
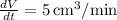`\( (dV)/(dt) = 5 \, \text{cm}^3/\text{min} \)`, and the volume
`\( V \)` of a sphere is
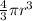`\( (4)/(3) \pi r^3 \)`, we can relate the rate of change of volume to the rate of change of the radius:

`\[ (dV)/(dt) = 4 \pi r^2 (dr)/(dt) \]`

Given
`\( (dV)/(dt) = 5 \, \text{cm}^3/\text{min} \) and \( r = 12 \, \text{cm} \), we can solve for \( (dr)/(dt) \):`

`\[ 5 = 4 \pi (12)^2 (dr)/(dt) \]`

Solving for
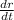`\( (dr)/(dt) \)` gives:

`\[ (dr)/(dt) = (5)/(4 \pi (12)^2) \]`

4. Now that we have
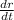`\((dr)/(dt)\)`, we can use it to find the rate of change of the surface area
`\((dA)/(dt)\)` using the formula:

`\[ (dA)/(dt) = 8 \pi r (dr)/(dt) \]`

Substitute the known values:

`\[ (dA)/(dt) = 8 \pi * 12 * (5)/(4 \pi (12)^2) \]`

`\[ (dA)/(dt) = (5)/(6) \, \text{cm}^2/\text{min} \]`

Therefore, the rate of change of the surface area when the radius is 12 cm is approximately
`\(0.833 \, \text{cm}^2/\text{min}\).`