Question

If 50 ml of 0.235 M NaCl solution is diluted to 200.0 ml what is the concentration of the diluted solution

Answer 1

The concentration of the diluted solution is 0.05875 M.

Step-by-step explanation:

To find the concentration of the diluted solution, we can use the formula:

M1V1 = M2V2

M1 is the initial concentration (0.235 M) and V1 is the initial volume (50 mL).

V2 is the final volume (200.0 mL) and we need to find M2, the final concentration.

Plugging in the values:

(0.235 M) * (50 mL) = M2 * (200.0 mL)

M2 = (0.235 M * 50 mL) / (200 mL) = 0.05875 M

So, the concentration of the diluted solution is 0.05875 M.

Answer 2

This is a straightforward dilution calculation that can be done using the equation

`M_1V_1=M_2V_2`

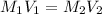
where M₁ and M₂ are the initial and final (or undiluted and diluted) molar concentrations of the solution, respectively, and V₁ and V₂ are the initial and final (or undiluted and diluted) volumes of the solution, respectively.

Here, we have the initial concentration (M₁) and the initial (V₁) and final (V₂) volumes, and we want to find the final concentration (M₂), or the concentration of the solution after dilution. So, we can rearrange our equation to solve for M₂:

`M_2=(M_1V_1)/(V_2).`

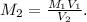
Substituting in our values, we get

`\[M_2=\frac{\left ( 50 \text{ mL} \right )\left ( 0.235 \text{ M} \right )}{\left ( 200.0 \text{ mL} \right )}= 0.05875 \text{ M}\].`

So the concentration of the diluted solution is 0.05875 M. You can round that value if necessary according to the appropriate number of sig figs. Note that we don't have to convert our volumes from mL to L since their conversion factors would cancel out anyway; what's important is the ratio of the volumes, which would be the same whether they're presented in milliliters or liters.