Question

Please help with this

Answer 1

Answer: with what part exactly

Explanation: I'm not sure what part you need help with

Answer 2

6.5 m

Step-by-step explanation:

See picture for working.

What we need to do is understand what's going on;

Initially, the speed and acceleration of the car, A, and the barge, B, and all the forces acting on them are 0;

Then the car moves across the barge 65 m;

This means it accelerates from 0 to a speed and moves 65 m in a certain time, t units of time;

Assuming firstly that there is no water resistance, as mentioned, and also no air resistance or friction as the car moves, then according to Newtons third law, a force will be exerted on the barge equal and opposite to the force moving the car forward;

So, we use the formula for force, F = ma, to set up 2 equations, one for the car and one for the barge :

Since the forces are equal, as established, we can solve them to get:

`a_(A) = 10a_(B)`

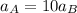
Next, we can formulate equations for the displacement of the car and barge:

s = ut + ¹/₂(at²)

(N.B. this formula assumes acceleration of the car and barge will be constant)

Then, setting up a ratio of the displacement of the car to that of the barge and then applying substitution, we get:

10 : 1

I.E. The displacement of the car is 10x that of the barge;

Since we know the displacement of the car, we can find the displacement of the barge.

Hope its clear.